Linear Speed Formula (Rotating Object)

The linear speed of a point on a rotating object depends on its distance from the center of rotation. The angular speed is the angle that an object moves through in a certain amount of time. The angular speed has units of radians per second (rad/s). There are 2π radians in a full circle. At a distance r from the center of the rotation, a point on the object has a linear speed equal to the angular speed multiplied by the distance r. The units of linear speed are meters per second, m/s.

linear speed = angular speed x radius of the rotation

v = ωr

v = linear speed (m/s)

ω = angular speed (radians/s)

r = radius of the rotation (m)

Linear Speed Formula (Rotating Object) Questions:

1) A power drill is on, and spinning at 10.0 revolutions per second (rev/s). The diameter of the drill bit is 4.00 mm. What is the linear speed of a point on the surface of the drill bit, in meters per second?

Answer: The first step is to find the angular speed of the drill bit. The revolutions per second must be converted to radians per second. There are 2π radians in a full circle.

ω = 10.0 rev/s

The distance between the center of rotation and a point on the surface of the drill bit is equal to the radius. The diameter of the drill bit is given, in units of millimeters. The radius in meters is,

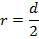
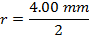
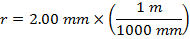
∴r = 0.002 m

Using the formula v = ωr, the linear speed of a point on the surface of the drill bit is,

v = ωr

∴v = (62.8 radians/s)(0.002 m)

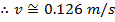
The linear speed of a point on the surface of the drill bit is approximately 0.126 m/s. Radians are a "placeholder" unit, and so they are not included when writing the solved value for linear speed.

2) Another question.

A sensor is connected inside a car wheel, which measures the linear speed. The sensor is 0.080 m from the center of rotation. At that position, the sensor reads that the linear speed of the wheel is 8.00 m/s. If the radius of the wheel is 0.220 m, what is the linear speed on the outer edge of the wheel?

Answer: The linear speed is different at different distances from the center of rotation, but the angular speed is the same everywhere on the wheel. To solve this problem, first find the angular speed using the linear speed at the position of the sensor, 0.080 m. The formula v = ωr can be rearranged to solve for the angular speed ω:

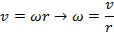
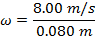
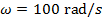
This is also the angular speed at the outer edge of the wheel, where the radius is r = 0.220 m. The formula v = ωr can be used again to solve for the linear speed at that radius:

v = ωr

v = (100 rad/s)(0.220 m)

∴v = 22.0 m/s

The linear speed of the car wheel at the outer edge is 22.0 m/s.