Relativity Formula

Special relativity states that time, length, energy, and momentum can depend on the velocity of one reference frame relative to another. An observer on a spaceship moving near the speed of light will measure time, length, energy, and momentum differently than an observer that is outside the ship. The formula that relates a value in one reference frame to the value in another is labeled with the Greek letter 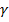("gamma"). It depends on the velocity, divided by the speed of light. The value 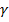is unitless.

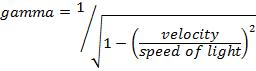
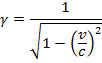
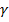= gamma, (unitless)

v = velocity (m/s)

c = speed of light ()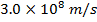

Relativity Formula Questions:

1) Imagine a clock on a spaceship. For an observer on the spaceship, standing next to the clock, the ticks of the clock happen at the same position. The clock is at rest relative to the observer. If the spaceship is moving, then according to an observer outside the ship, the ticks of the clock happen at different positions. The passage of time according to the person on the ship is called one-position time or proper time, and is labeled . The two-position time or observer time is the time observed by the person outside the ship, and is labeled . The relationship between one-position and two-position time is 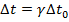. If a spaceship is moving at 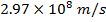, and the observer on the ship measures that 30.0 seconds have passed, what is the value of 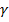, and how much time will have passed according to the observer outside the ship?

Answer: The value of 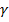can be found using the formula:

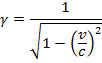
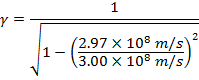
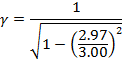
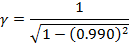
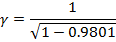
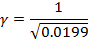
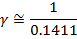
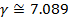
The value of 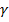is 7.089. The amount of time that passes inside the ship is the proper time, 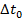. The time that passes outside the ship can be found from 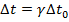. When 30.0 s passes inside the ship, the time that passes outside the ship is:

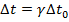
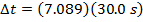
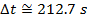
Approximately 212.7 seconds pass outside the ship.

2) The distance between two points, and therefore the length of objects, depends on who is measuring. Imagine a person on a spaceship. The ship is at rest relative to the person. In this reference frame, the length of the ship is called the proper length, and is labeled 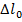. Outside the ship, an observer will see the ship moving. In this reference frame, the length of the ship will be different. The observed length is labeled 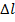. The observed length (outside the ship) is always shorter than the proper length (inside the ship). The relationship between proper length and observed length is 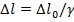. If a spaceship is moving at 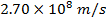, and the observer on the ship measures the length of the ship to be 700.0 m (meters), what is the value of 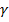, and what will an observer outside the ship measure the length to be?

Answer: The value of 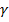can be found using the formula:

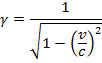
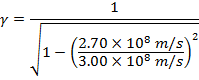
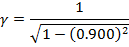
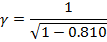
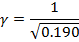
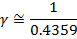
The value of 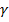is 2.294. The length of the ship as measured by the person on board is the proper length, 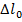. The length of the ship measured by the outside observer can be found from 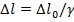. If the proper length of the ship is 700.0 m, the length according to the outside observer is:

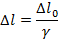
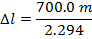
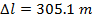
The length of the ship according to the outside observer is approximately 305.1 meters.